Mathematics Of Finance Simple Interest Rate Ex 5 1 Q 15 Saima Academy English Youtube About press copyright contact us creators advertise developers terms privacy policy & safety how works test new features nfl sunday ticket press copyright. About press copyright contact us creators advertise developers terms privacy policy & safety how works test new features nfl sunday ticket press copyright.
Chapter 3 Mathematics Of Finance Section 1 Simple Interest I = prn. alternatively, you can use the simple interest formula i=prn if you have the interest rate per month. if you had a monthly rate of 5% and you'd like to calculate the interest for one year, your total interest would be $10,000 × 0.05 × 12 = $6,000. the total loan repayment required would be $10,000 $6,000 = $16,000. The annual interest rate on the loan is 6%. the term of the loan is three years. using the simple interest formula above, the amount of simple interest on the student's loan is: \begin {aligned. Chapter outline: this chapter will provide readers with a comprehensive understanding of simple interest, including. 1.1 interest, principal, time: this section introduces the basic concept of simple interest and explains how to calculate the interest amount, interest rate, and time. 1.2 future and present values: this section delves into the. So a = 3000(1 0.06 12)20 × 12 = $9930.61 (round your answer to the nearest penny) let us compare the amount of money earned from compounding against the amount you would earn from simple interest. years. simple interest ($15 per month) 6% compounded monthly = 0.5% each month. 5.
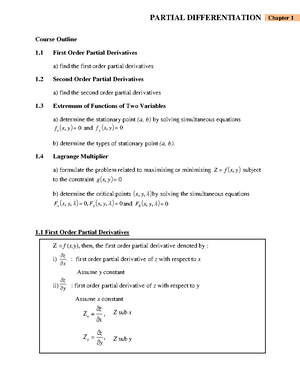
Chapter 7 Mathematics Of Finance Mathematics Of Finance 7 Simple Interest Find The Simple Chapter outline: this chapter will provide readers with a comprehensive understanding of simple interest, including. 1.1 interest, principal, time: this section introduces the basic concept of simple interest and explains how to calculate the interest amount, interest rate, and time. 1.2 future and present values: this section delves into the. So a = 3000(1 0.06 12)20 × 12 = $9930.61 (round your answer to the nearest penny) let us compare the amount of money earned from compounding against the amount you would earn from simple interest. years. simple interest ($15 per month) 6% compounded monthly = 0.5% each month. 5. The rate of interest is given as a percentage per year, expressed as a decimal. for example, 6% = 0.06 and 11 1 2 % = 0.115. the time the money is earning interest is calculated in years. one year’s interest is calculated by multiplying the principal times the interest rate, or pr. if the. Of special importance is the interest rate per period, denoted i, which is calculated by dividing the annual interest rate by the number of interest periods per year. for example, in our statement in table 1, the annual interest rate is 4%, the interest is com pounded quarterly, and the interest rate per period is 4%>4 =.04 4 = .01.
Module 5 Mathematics Of Finance Chapter 5 Mathematics Of Finance Introduction Mathematics And The rate of interest is given as a percentage per year, expressed as a decimal. for example, 6% = 0.06 and 11 1 2 % = 0.115. the time the money is earning interest is calculated in years. one year’s interest is calculated by multiplying the principal times the interest rate, or pr. if the. Of special importance is the interest rate per period, denoted i, which is calculated by dividing the annual interest rate by the number of interest periods per year. for example, in our statement in table 1, the annual interest rate is 4%, the interest is com pounded quarterly, and the interest rate per period is 4%>4 =.04 4 = .01.
Lecture 05 Financial Mathematics Interest Rates Lecture 5 Financial Mathematics Interest
Comments are closed.